the general equation of a graph is y= mx + c
m is the gradient and c is the intercept
from the first line equation, m = 1/3
condition for perpendicularity is that if two lines are perpendicular
the gradient of the first line is equal to the negative of the reciprocal of the second line gradient
so
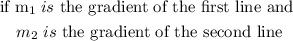
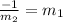
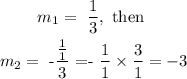

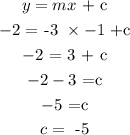
so the equation of the line is obtain by substituting the values of the gradient (m2) and the intercept (c) in y = mx +c
so the equation is y = -3x + (-5)
y = -3x -5