Question

Sketch trapezoid HJKL with vertices H(–4, 2), J(1, 7), K(8, 4), and L(–1, –5) on graph paper, and then answer the following questions: (a) Name the parallel sides. (b) What are the slopes of the parallel sides? (c) What are the lengths of the non-parallel sides? (d) Is the trapezoid an isosceles trapezoid?

Answer 1

The coordinates of the trapezoid HJKL with vertices

H(-4,2)

J(1,7)

K(8,4)

L(-1,-5)

Plot these coordinates on the graph.

(a). The parallel lines are the pair of lines which never intersect.

In the given figure the parallel sides are HJ and KL

HJ and KL are parallel side.

(b). Slope is define as the ration of rate of change in y coordinates to the rate in change in y coordinates

`\text{slope}=(y_2-y_1)/(x_2-x_1)`

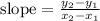
The slope of parallel lines are always equal

so, slope of HJ=slope of KL,

For the slope of HJ,

The coordinates are H(-4, 2) J(1,7)

`\begin{gathered} x_1=-4,x_2=1,y_1=2,y_2=7 \\ \text{Substitute the values in the equation of slope} \\ \text{Slope}=(7-2)/(-4-1) \\ \text{Slope}=(5)/(-5) \\ \text{Slope}=(-1) \end{gathered}`

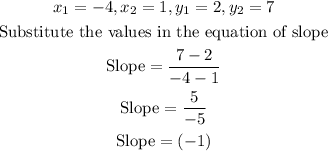
Slope of HJ is (-5) so, the slope of KL=(-1)

SLOPE of HJ=KL=(-5).

(c). For the length of parallel side, use the distance formula to find the distance between teh coordinates.

The non parallel sides are : HL and JK

Distance formula is expressed as :

`\text{Distance}=\text{ }\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}`

for the length of HL, the coordinates are H(-4,2) & L(-1,-5)

`\begin{gathered} x_1=-4,x_2=-1,y_1=2,y_2=-5 \\ \text{substitute the value in the distance formula} \\ HL=\sqrt[]{(-1-(-4))^2+(-5-2)^2} \\ HL=\sqrt[]{(-1+5)^2+(-7)^2} \\ HL=\sqrt[]{4^2+(-7)^2} \\ HL=\sqrt[]{16+49} \\ HL=\sqrt[]{65} \\ HL=8.06\text{ unit} \end{gathered}`

Now, for the length of JK, the coordinates are J(1,7) & K(8,4)

`\begin{gathered} x_1=1,x_2=8,y_1=7,y_2=4 \\ \text{substitute the value in the distance formula} \\ JK=\sqrt[]{(8-1)^2+(4-7)^2} \\ JK=\sqrt[]{(7)^2+(-3)^2} \\ JK=\sqrt[]{49+9^{}} \\ JK=\sqrt[]{58} \\ JK=7.61\text{ unit} \end{gathered}`

The length of non parallel sides are HL=8.06 unit & JK=7.61 unit.

(d). A trapezoidal is said to be issoceles trapezoidal if the base angles are equal and there for left and right sides are equal,

since from the above calculation.,

`HL\\e JK`

So, the trapezoidal is not issoceles.

ANSWER :

(a). HJ and KL are parallel side.

(b). Slope of HJ=KL=(-5).

(c). Length of non parallel sides are HL=8.06 unit & JK=7.61 unit.

(d). The trapezoidal is not issoceles.