We'll use a compound rule of three to solve your problem:
3 gangs 20km 10 days
x gangs 50 km 15 days
Gangs are directly proportional to the surface and indirectly proportional to the number of days:
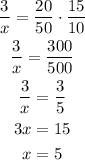
So, it's needed 5 gangs to complete the work, in total.