Okay, here we have this:
Considering the provided equation, we will identify if its roots are negative, positive or imaginary, so we obtain the following:
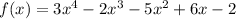
According to the rule of the signs of Descartes, we can identify that since in f(x) there are three changes of signs then there will be 1 or 3 positive roots.
Now, let's find p(-x) to see how many possible negative roots there are:
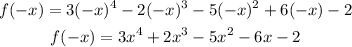
Since there is only one sign change, then there is only one negative root.
Now, finally we will factor it to see how many positive roots we can get:
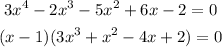
As it cannot be simplified further, we finally obtain that f (x) has a positive root, a negative root and two imaginary ones.