Question

Given a and b are the first-quadrant angles, sin a=5/13, and cos b=3/5, evaluate sin(a+b)

Answer 1

63/65

Explanation:

Step 1

Given that a and b are first-quadrant angles. In addition:

`\begin{gathered} \sin a=(5)/(13) \\ \cos b=(3)/(5) \end{gathered}`

Using the double-angle formula:

`\sin (a+b)=\sin a\cos b+\cos a\sin b`

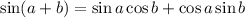
Step 2

We need to find the values of cos a and sin b.

(i)cos a

From trigonometric ratios:

`\begin{gathered} \sin \theta=\frac{\text{Opposite}}{\text{Hypotenuse}} \\ \sin a=(5)/(13)\implies\text{Opp}=5,\text{Hyp}=13 \end{gathered}`

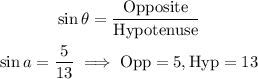
We find the length of the adjacent side using the Pythagorean Theorem.

`\begin{gathered} \text{Hyp}^2=\text{Opp}^2+\text{Adj}^2 \\ 13^2=5^2+\text{Adj}^2 \\ \text{Adj}^2=13^2-5^2=144=12^2 \\ \text{Adj}=12 \end{gathered}`

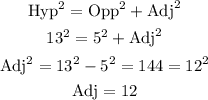
Therefore:

`\begin{gathered} \cos \theta=\frac{\text{Adjacent}}{\text{Hypotenuse}} \\ \cos a=(12)/(13) \end{gathered}`

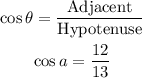
(b) sin b

From trigonometric ratios:

`\begin{gathered} \cos \theta=\frac{\text{Adjacent}}{\text{Hypotenuse}} \\ \cos b=(3)/(5)\implies\text{Adj}=3,\text{Hyp}=5 \end{gathered}`

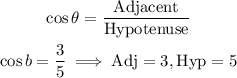
We find the length of the opposite side using the Pythagorean Theorem.

`\begin{gathered} \text{Hyp}^2=\text{Opp}^2+\text{Adj}^2 \\ 5^2=\text{Opp}^2+\text{3}^2 \\ \text{Opp}^2=5^2-3^2=25-9=16 \\ \text{Opp}=\sqrt[]{16}=4 \end{gathered}`

Therefore:

`\begin{gathered} \sin \theta=\frac{\text{Opposite}}{\text{Hypotenuse}} \\ \sin b=(4)/(5) \end{gathered}`

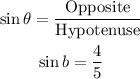
Step 3

Substitute the values of cos a and sin b into the double angle formula.

`\begin{gathered} \sin (a+b)=\sin a\cos b+\cos a\sin b \\ =(5)/(13)*(3)/(5)+(12)/(13)*(4)/(5) \\ =(15)/(65)+(48)/(65) \\ =(63)/(65) \end{gathered}`

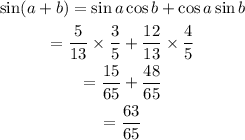
The value of sin(a+b) is 63/65.