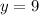Answer:
The distance between L and the line KH and HF is;

The value of y is;
Step-by-step explanation:
Given the figure in the attached image;
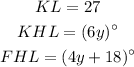
From the image;
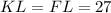
So, the distance between L and the line KH and HF is;

Also, the triangles KHL and FHL are congruent.
So, the angles KHL and FHL are congruent.
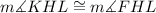
Substituting the expressions;
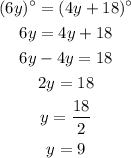
Therefore, the value of y is;