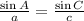Question

Given: BD⎯⎯⎯⎯⎯ is an altitude of △ABC.Prove: sinAa=sinCcTriangle A B C with an altitude B D where D is on side A C. side A C is also labeled as small b. Side A B is also labeled as small c. Side B C is also labeled as small a. Altitude B D is labeled as small h. Select from the drop-down menus to correctly complete the proof.Statement ReasonBD⎯⎯⎯⎯⎯ is an altitude of △ABC. Given△ADB and △CDB are right triangles. Definition of right trianglesinA=hcand sinC=hacsinA=hand asinC=hMultiplication Property of EqualitycsinA=asinCcsinAac=asinCacsinAa=sinCcSimplify.

Answer 1

EXPLANATION :

From the problem, triangles ADB and CDB are right triangles.

In a right triangle, the sine function is the opposite side divided by the hypotenuse.

Referring to the figure,

sin A = h/c and sin C = h/a which are both definitions of sine ratio.

So the reason is Definition of sine ratio

By multiplication property of equality, use cross multiplication to remove the denominators

That's

c sinA = h

and

a sin C = h

Equating both equations in h :

c sin A = a sin C by the reason of Substitution Property of Equality

Diving both sides by "ac"

`(c\sin A)/(ac)=(a\sin C)/(ac)`

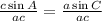
by the reason of Division Property of Equality

Then :

`(\sin A)/(a)=(\sin C)/(c)`