For the given equation;
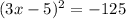
We shall begin by expanding the parenthesis on the left side, after which we would combine all terms on and move all of them to the left side, which shall yield a quadratic equation. Then we shall solve.
Let us begin by expanding the parenthesis;
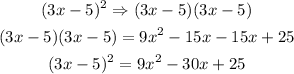
Now that we have expanded the left side of the equation, we would have;
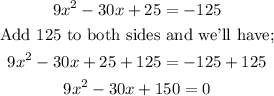
We shall now solve the resulting quadratic equation using the quadratic formula as follows;
![\begin{gathered} x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ \text{Where;} \\ a=9,b=-30,c=150 \\ x=\frac{-(-30)\pm\sqrt[]{(-30)^2-4(9)(150)}}{2(9)} \\ x=\frac{30\pm\sqrt[]{900-5400}}{18} \\ x=\frac{30\pm\sqrt[]{-4500}}{18} \\ x=\frac{30\pm\sqrt[]{-900*5}}{18} \\ x=\frac{30\pm\sqrt[]{-900}*\sqrt[]{5}}{18} \\ x=\frac{30\pm30i\sqrt[]{5}}{18} \\ \text{Therefore;} \\ x=\frac{30+30i\sqrt[]{5}}{18},x=\frac{30-30i\sqrt[]{5}}{18} \\ \text{Divide all through by 6, and we'll have;} \\ x=\frac{5+5i\sqrt[]{5}}{3},x=\frac{5-5i\sqrt[]{5}}{3} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/mhyx213miiua9dhgwmyv41un27qdfsc30t.png)
ANSWER:
![x=\frac{5+5i\sqrt[]{5}}{3},x=\frac{5-5i\sqrt[]{5}}{3}](https://img.qammunity.org/2023/formulas/mathematics/college/7im7dedghkngb3w2ilxxxbzub9yaswadet.png)