Question

**Which of the following represents the sum of all possible solutions to the equation below?(18 - 3w)/(w+6)=w^2/(w+6)(A) -9(B) -3(C) 3(D) 9

Answer 1

We need to solve the given equation and then find the sum of all possible solutions.

The equation is:

`(18-3w)/(w+6)=(w^2)/(w+6)`

Notice that the denominator on both sides is w+6. Since the denominator can't be zero, we have:

`\begin{gathered} w+6\\e0 \\ \\ w\\e-6 \end{gathered}`

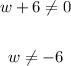
Thus, -6 can't be a solution.

Now, we can solve the equation by rewriting it as

`\begin{gathered} (18-3w)/(w+6)\cdot(w+6)=(w^2)/(w+6)\cdot(w+6) \\ \\ 18-3w=w^(2) \\ \\ w^(2)+3w-18=0 \end{gathered}`

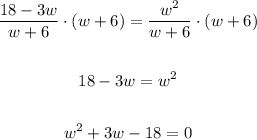
Now, we can use the quadratic formula to solve it:

`\begin{gathered} w=\frac{-3\pm\sqrt[]{3^(2)-4(1)(-18)}}{2(1)} \\ \\ w=\frac{-3\pm\sqrt[]{9+72}}{2} \\ \\ w=\frac{-3\pm\sqrt[]{81}}{2} \\ \\ w=(-3\pm9)/(2) \\ \\ w_1=(-3-9)/(2)=-6\text{ (this solution is not possible)} \\ \\ w_2=(-3+9)/(2)=3 \end{gathered}`

Therefore, the only possible solution is 3. And the sum of all possible solutions is 3.