Step 1
Given;
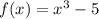
Required; To find the inverse of f(x)
Step 2
Find the inverse
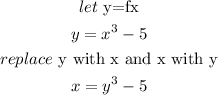
Then solve for y
![\begin{gathered} x=y^3-5 \\ y^3=x+5 \\ Take\text{ cube root of both sides} \\ \sqrt[3]{y^3}=\sqrt[3]{x+5} \\ y=\sqrt[3]{x+5} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/bagzntz6hzqpgc4r4abu3c0wkkecj2j6sw.png)
Hence,
![f^(-1)(x)=\sqrt[3]{x+5}](https://img.qammunity.org/2023/formulas/mathematics/college/k27xdsfqyl04xzr0fggcm87puaw4p77hjp.png)
Step 3
Choose 3 points on f(x) and use these points to show that the inverse is correct.
The 3 points are;
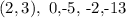
![\begin{gathered} f^(-1)(x)=\sqrt[3]{x+5} \\ (2,3),\text{ where for inverse x=3, y=2} \\ f^(-1)(x)=\sqrt[3]{3+5} \\ f^(-1)(x)=2 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/iqoytwchbp6xj8wydj2bqfpa6mpaglmcuo.png)
![\begin{gathered} f^(-1)(x)=\sqrt[3]{x+5} \\ (0,-5) \\ f^(-1)(x)=\sqrt[3]{-5+5} \\ f^(-1)(x)=0 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/1e35lhgw6m3lxa42hfb119c3qox7k0ixz2.png)
![\begin{gathered} f^(-1)(x)=\sqrt[3]{(x+5)} \\ (-2,-13) \\ f^(-1)(x)=\sqrt[3]{-13+5} \\ f^(-1)(x)=\sqrt[3]{-8}=-\sqrt[3]{8}=-2 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/mekw8tonrrnjqm60qc30nqgba17ih66vve.png)
Hence, having seen that when we substitute y for x from the points from f(x), we get x for y from f(x), the inverse is correct.