Question

Mr.Spence built a rectangular garden surrounded by a walkway. The walkway is an equal width around the whole garden.What is the area of the garden?A. 600 square feetB. 336 square feetC. 459 square feetD. 1,800 square feet

Answer 1

B. 336 square feet

Step-by-step explanation:

The larger rectangle has a width of 20ft and a length of 30ft.

Width of the walkway = 3 feet.

Since the walkway is around the garden, we subtract the width on both sides.

Therefore:

`\begin{gathered} \text{The length of the garden }=30-(3+3) \\ =30-6 \\ =24ft \end{gathered}`

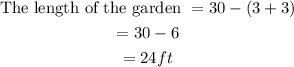
Similarly:

`\begin{gathered} \text{The width of the garden }=20-(3+3) \\ =20-6 \\ =14ft \end{gathered}`

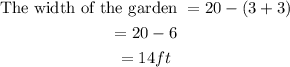
We then calculate the area of the garden.

`\begin{gathered} \text{Area of the garden=Length}* Width \\ =24*14 \\ =336\text{ square feet} \end{gathered}`

The area of the garden is 336 square feet.