ANSWER
The vertex is (-7, 4)
Step-by-step explanation
We usually see the equation of a parabola with this form:

Where (h, k) is the vertex of the parabola.
But in this problem, the variables are changed, so we're looking for an equation like:

The vertex is also point (h, k).
To complete the square we have to see the terms that contain y: y² - 8y
We know that a perfect square has the form:

If the term that contains y with exponent 1 has a coefficient 8, then we can find the second number of the binomial for the perfect square:
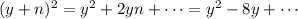
let's not think of the third term just yet. For now we have to concentrate on the second term:

The second number for the perfect square is -4:
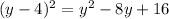
Now we have to put this inside the equation. Since now we have a third term that is 16 we have to subtract 16 so we keep the equation true. I'll replace y²-8y by (y-4)²-16:

We can add 4x on both sides so we have the x and y separate:

And finally we have to divide both sides by 4:
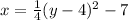
So in this case k = 4 and h = -7. The vertex is (-7, 4)