In order to calculate the translation of <-4, -1> to the triangle ABC, we just need to add these coordinates to all vertices of the triangle, that is, add -4 to the x-coordinate and -1 to the y-coordinate. So we have that:
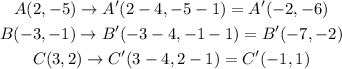
So the vertices after the translation are A'(-2, -6), B'(-7, -2) and C'(-1, 1).