Question

The following is a sample of 20 measurements.Answer b part

Answer 1

b)

Given:

`\begin{gathered} \bar{x}=10.2 \\ s=2.12 \end{gathered}`

Hence,

`\begin{gathered} \bar{x}\pm s=10.2\pm2.12 \\ \bar{x}+s=12.32 \\ \bar{x}-s=8.08 \end{gathered}`

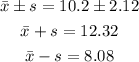
So, the measurements in the data between 8.08 and 12.32 are 11, 9, 12, 10 12, 12 , 12, 9, 9, 9, 11, 11, 12 and 11.

Therefore, the number of measurements in interval x±s is 14.

The percentage of the measurements that fall between the interval x±s is,

`\text{Percent}=(14)/(20)*100=70`

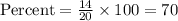
Therefore, the percentage of the measurements that fall between the interval x±s is 70%.

Now,

`\begin{gathered} \bar{x}\pm2s=10.2\pm2*2.12 \\ \bar{x}\pm2s=10.2\pm4.24 \\ \bar{x}+2s=14.44 \\ \bar{x}-2s=5.96 \end{gathered}`

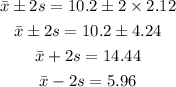
So, all the measurements in the data are between 5.96 and 14.44.Therefore, the number of measurements in interval x±2s is 20.

Therefore, the percentage of the measurements that fall between the interval x±2s is 100%.

Now,

`\begin{gathered} \bar{x}\pm3s=10.2\pm3*2.12 \\ \bar{x}\pm3s=10.2\pm6.36 \\ \bar{x}+3s=16.56 \\ \bar{x}-3s=3.84 \end{gathered}`

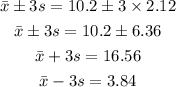
So, all the measurements in the data are between 3.84 and 16.56.Therefore, the number of measurements in interval x±3s is 20.

Therefore, the percentage of the measurements that fall between the interval x±3s is 100%.

Last part: compare the percentage .

According to empirical rule, approximately 68% of the measurements in a sample will fall within the interval x±s.

From part b, the obtained percentage of measurements that fall within the interval x±s is 70%.

Therefore, percentage of measurements that fall within the interval x±s is greater than the predicted percentage for x±s using the empirical rule.

Option C is correct.