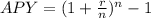To calculate the ampount of money in the account after 5 years, we will use the formula:
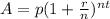
where A is the final amount
P is the initial amount or principal
r is the rate
n is the number of times the interest is applied
t is the time in years
From the question,
P = $6000 r = 2.12/100 = 0.0212 t= 5 n=365
substitute the values ibto the formula and evaluate

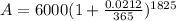
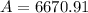
The amount is $6670.91
b)
To find the annual percentage yield (APY) on this account, we will use the formula: