Question

A racing car of mass 1500 kg, is accelerating at 5.0 m/s2, is experiencing a lift force of 600 N [up}, due to its streamlined shape, and grounding effects of 1000 N [down], due to air dams and spoilers. Find the driving force needed to keep the car going given that μk = 1.0.

Answer 1

Given data:

* The acceleration of the car is,

`a=5ms^(-2)`

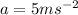
* The mass of the car is,

`m=1500\text{ kg}`

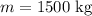
* The force acting on the car in the upward direction is,

`F_1=600\text{ N}`

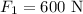
* The force acting on the car in the downward direction is,

`F_2=1000\text{ N}`

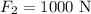
* The coefficient of kinetic friction is,

`\mu_k=1`

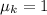
Solution:

The weight of the car is,

`\begin{gathered} w=mg \\ w=1500*9.8 \\ w=14700\text{ N} \end{gathered}`

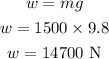
The normal force acting on the car is,

`\begin{gathered} F_N=w+F_2-F_1 \\ F_N=14700+1000-600 \\ F_N=15100\text{ N} \end{gathered}`

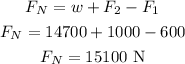
The frictional force acting on the car is,

`\begin{gathered} F_k=\mu_kF_N \\ F_k=1*15100 \\ F_k=15100\text{ N} \end{gathered}`

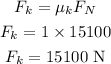
According to newton's second law, the force acting on the car is,

`\begin{gathered} F=ma \\ F=1500*5.0 \\ F=7500\text{ N} \end{gathered}`

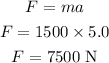
The net force acting on the car in terms of the applied force and frictional force is,

`\begin{gathered} F=F_a-F_k \\ F_a=F+F_k \end{gathered}`

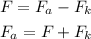
Substituting the known values,

`\begin{gathered} F_a=7500+15100 \\ F_a=22600\text{ N} \end{gathered}`

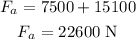
Thus, the driving force required to maintain the motion of the car is 22600 N.