Step-by-step explanation:
To solve the question, we will need to re-express the given function as follow:
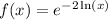
Will become

Thus
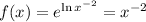
This simply means that we will find the area under the curve:
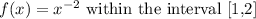
Thus
The area will be
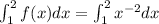
This will then be
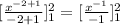
This will be simplified to give
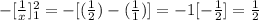
Therefore, the area under the curve will be
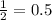
Thus, the answer is 0.5