Answer:
10. The triangles are similar.
11. The slope of the line is
 .
.
12. The given equation is incorrect.
13. They have the same slope.
Explanation:
Hi there!
---
These two triangles are similar. They share angle QPT, and angles PTQ and PSR are have the same measure as well. Because these triangles share two congruent angles, they are therefore similar.
This is the AA similarity theorem, which states that if two angles in one triangle are the same as two angles of another, the two triangles are therefore similar.
---
Points P and R both fall onto the line on the graph. The slope of a line is equal to its "rise over run", or "the number of units the line travels up" over "the number of units the line travels to the right".
Notice how, on the graph, for every 2 units the line travels up, it travels 3 units to the right.
Therefore, the slope of the line is 2 over 3, or
 .
.
---
Linear equations are typically organized in slope-intercept form, which looks like this:
 where m is the slope and b is the y-intercept, or the value of y when the line crosses the y-axis.
where m is the slope and b is the y-intercept, or the value of y when the line crosses the y-axis.
In the given equation
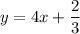 , the slope and the y-intercept have been switched. Therefore, this is an incorrect equation.
, the slope and the y-intercept have been switched. Therefore, this is an incorrect equation.
---
These two lines both fall onto the same line that's been graphed on the same plane. Therefore, yes, they have the same slope.
I hope this helps!