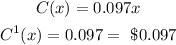To solve for the total cost of producing the following numbers of cups of coffee:
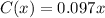
where
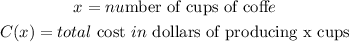
(a) The total cost of producing 1000 cups =
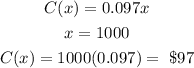
(b) The total cost of producing 1001 cups =
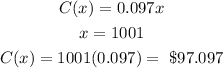
(c) The marginal cost for any cup = $0.097
marginal cost can be found by taking the derivative of the function