We have the rational function:
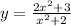
and we have to find points of discontinuity.
This points happen when the denominator becomes 0, because the function became undefined in those cases.
In this case it would happen when:
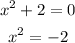
As there is no real value for x that makes the square of x be a negative number, we don't have points of discontinuity for this function.
NOTE: x^2+2 has two complex roots and never intercepts the x-axis.
Answer: this function has no points of discontinuity.
Check for asymptotes.
As there are no discontinuities, we don't have vertical asymptotes.
We will check if there are horizontal asymptotes:
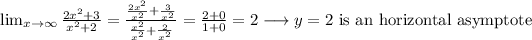
If we repeat for minus infinity (the left extreme of the x-axis), we also get y=2.