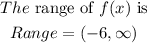Notice that,
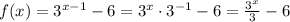
And there are no restrictions for the values that x can take. The domain is the whole set of real numbers.
Now, we need to check for the limits when x->+/- infinite, as follows:
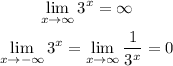
Then, the range of 3^x is (0, infinite).
Finally, we can get the range of function f(x):
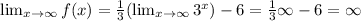
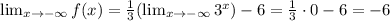
Then,