Answer:
The solution to the system of equations is
x = 3
y = 4
Step-by-step explanation:
Given the pair of equations:

To solve these simultaneously, use the expression for x in equation (1) in equation (2)

Using y = 4 in equation (1)
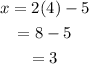
Therefore, x = 3, and y = 4