Given the inequality:
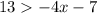
• You can solve it as follows:
1. Apply the Addition Property of Inequality by adding 7 to both sides of the inequality:
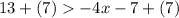

2. Apply the Division Property of Inequality by dividing both sides of the inequality by -4 (since you are dividing both sides by a negative number, the direction of the inequality symbol changes):
![\begin{gathered} (20)/(-4)<(x)/(-4) \\ \\ -53. You can rewrite the solution in this form:[tex]x>-5]()
• In order to graph the solution on the Number Line, you can follow these steps:
1. Since the symbol is:
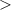
You need to draw an open circle over the number -5.
2. Draw a line from the circle to the right.
Then, you get:
Hence, the answer is:
• Solution:

• Number Line: