The annual percentage yield is given by the following formula:
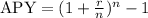
Where r is the stated annual interest rate (in decimal form) and n is the number of times compounded.
A) APY for money invested at an annual rate of 4.09% compounded monthly.
Thus, the annual interest rate in decimal form is:
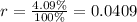
And as it is compounded monthly then n=12.
Replace these values and solve:
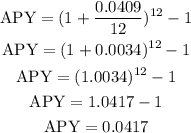
The APY is 0.0417=4.17%.
B) 4.1% compounded quarterly:
The annual interest rate is:

As it is compounded quarterly then n=4.
Replace and solve:

The APY is 0.1302=13.02%