Question

Evaluate: sin(30 degrees)cos(60 degrees)=(See attached image to assist with these 2 problems)

Answer 1

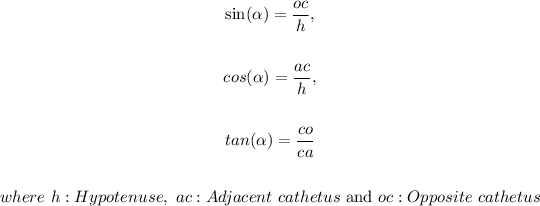
According to the figure we need to evaluate the sin(30°) and the cos(60°). Remember the trigonometric relations defined over the rectangle triangles as follows, suppose we have an angle called "alpha"

`\begin{gathered} \sin(\alpha)=(oc)/(h), \\ \\ cos(\alpha)=(ac)/(h), \\ \\ tan(\alpha)=(co)/(ca) \\ \\ where\text{ }h:Hypotenuse,\text{ }ac:Adjacent\text{ }cathetus\text{ and }oc:Opposite\text{ }cathetus \end{gathered}`

Now, according to the figure, we have that for the angle of 60 degrees:

`\begin{gathered} h=2x,ac=x,oc=√(3)x \\ \\ \sin(60°)=(oc)/(h)=(√(3)x)/(2x)=(√(3))/(2) \\ \\ \cos(60^(\circ))=(ac)/(h)=(x)/(2x)=(1)/(2) \end{gathered}`

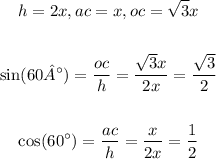
And for the angle of 30 degrees we get the following

`\begin{gathered} h=2x,oc=x,ac=√(3)x \\ \\ \sin(30°)=(oc)/(h)=(x)/(2x)=(1)/(2)=\cos(60°) \\ \\ \cos(30^(\circ))=(ac)/(h)=(√(3)x)/(2x)=(√(3))/(2)=\cos(60^(\circ)) \end{gathered}`

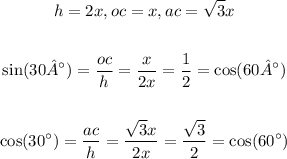
So, your answer is: sin(30°)=1/2=cos(60°).