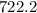Question

A mandatory competency test for high school sophomores has a normal distribution with a mean of 553 and a standard deviation of 90. The top 3% of students receive a $500 prize. What is the minimum (cutoff) score you would need to receive this award?

Answer 1

The minimum (cut off) score you need to receive this award is;

`722.2`

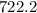
Step-by-step explanation:

Given that the top 3% of the students receive a $500 prize.

`P=1-0.03=0.97`

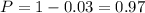
We will then find the z-score that corresponds to the given probability.

`z=1.88`

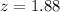
Recall that;

`z=(X-\mu)/(\sigma)`

Given;

`\begin{gathered} \mu=553 \\ \sigma=90 \end{gathered}`

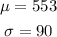
substituting the values;

`\begin{gathered} 1.88=(X-553)/(90) \\ X-553=1.88*90 \\ X=553+1.88*90 \\ X=722.2 \end{gathered}`

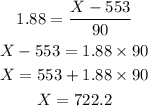
Therefore, the minimum (cut off) score you need to receive this award is;

`722.2`