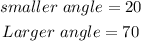Question

Three support beams for a bridge form a pair of complementary angles. Find the measure of each angle.(2x - 20)INNISmaller angle:Larger angle:O0UNSFANA(4x - 10)

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: Define complementary angles

When the sum of two angles is 90°, then the angles are known as complementary angles.

STEP 2: Write the pairs of the angles formed

`\begin{gathered} (2x-20)\degree \\ (4x-10)\degree \end{gathered}`

STEP 3: find the value of x

Following the definition in step 1, this goes that;

`\begin{gathered} (2x-20)+(4x-10)=90\degree \\ 2x-20+4x-10=90\degree \\ 6x-30=90 \\ 6x=90+30 \\ 6x=120 \\ x=(120)/(6)=20 \end{gathered}`

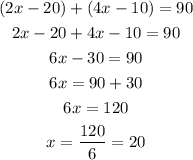
STEP 4: Find the two pair of angles

`\begin{gathered} (2x-20)=2(20)-20=40-20=20\degree \\ 4x-10=4(20)-10=80-10=70\degree \end{gathered}`

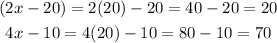
Therefore,

`\begin{gathered} smaller\text{ }angle=20\degree \\ Larger\text{ }angle=70\degree \end{gathered}`