Question

Write an equation in slope-intercept form that contains the points (2, 8) and (4, 9).

Answer 1

Given two points, the equation of the line in slope form can be obtained using this equation

`(y_2-y_1)/(x_2-x_1)\text{ = }\frac{y_{}-y_1}{x_{}-x_1}`

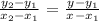
Now we can name the points

x1 = 2, y1 = 8

x2 = 4 , y2 =9

These coordinates can then be substituted into the equation

`(9-8)/(4-2)\text{ =}\frac{y\text{ - 8}}{x\text{ - 2}}`

`\begin{gathered} (1)/(2)\text{ = }\frac{y\text{ - 8}}{x\text{ - 2}} \\ \\ x-2\text{ = 2 (y - 8)} \\ \\ x\text{ - 2 = 2y - 16} \end{gathered}`

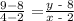
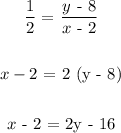
x - 2 + 16 = 2y

2y = x - 2 +16

2y = x + 14

Divide both sides by 2

y = x/2 + 14/2

`y\text{ = }(x)/(2)\text{ + 7}`

This is the equation in slope-intercept form

where the slope = 1/2