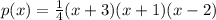Since the polynomial must have zeroes at x=-3, x=-1, x=2, then, we can write it as a combination of the factors (x+3), (x+1), (x-2):
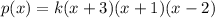
The constant k will help us to adjust the value of the polynomial when x=3:
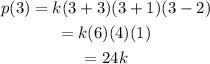
Since p(3) must be equal to 6, then:
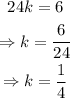
Therefore, the following polynomial function has zeroes at -3, -1 and 2, and passes through the point (3,6):