Question

Write an equation in general form of the circle with the given properties. Ends of diameter at (5,7) and (-5,-7) ?

Answer 1

Write an equation in general form of the circle with the given properties.

Ends of diameter at (5,7) and (-5,-7)

we have that

the equation of the circle is

(x-h)^2+(y-k)^2=r^2

where (h,k) is the center and r is the radius

step 1

Find the center

Remember that

The center of the circle is the midpoint of diameter

so

`\begin{gathered} (h,k)=((5-5)/(2),(7-7)/(2)) \\ (h,k)=(0,0) \end{gathered}`

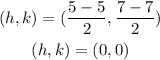
the center is the origin

step 2

Find the radius

Find the diameter

calculate the distance between two points

`\begin{gathered} d=\sqrt[\square]{(-7-7)^2+(-5-5)^2} \\ d=\sqrt[\square]{(-14)^2+(-10)^2} \\ d=\sqrt[\square]{296} \end{gathered}`

simplify

`D=\sqrt[\square]{296}=2\sqrt[\square]{74}`

the radius is half the diameter

so

r=2√74/2=√74

step 3

the equation iof the circle is

x^2+y^2=(√74)^2

x^2+y^2=74