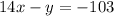Question

find an equation of the line passing through the pair points. write the equation in the form ax+by=c (-7,5),(-8,-9)

Answer 1

Given the pair of coordinates;

`\begin{gathered} (-7,5) \\ (-8,-9) \end{gathered}`

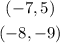
We would begin by first calculating the slope of the line.

This is given by the formula;

`m=(y_2-y_1)/(x_2-x_1)`

The variables are as follows;

`\begin{gathered} (x_1,y_1)=(-7,5) \\ (x_2,y_2)=(-8,-9) \end{gathered}`

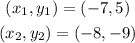
We will now substitute these into the formula for finding the slope as shown below;

`\begin{gathered} m=((-9-5))/((-8-\lbrack-7)) \\ \end{gathered}`
`\begin{gathered} m=(-14)/(-8+7) \\ \end{gathered}`
`\begin{gathered} m=(-14)/(-1) \\ m=14 \end{gathered}`

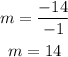
The slope of this line equals 14. We shall use this value along with a set of coordinates to now determine the y-intercept.

Using the slope-intercept form of the equation we would have;

`y=mx+b`

We would now substitute for the following variables;

`\begin{gathered} m=14 \\ (x,y)=(-7,5) \end{gathered}`
`5=14(-7)+b`
`5=-98+b`

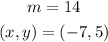
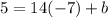
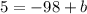
Add 98 to both sides of the equation;

`103=b`

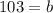
We now have the values of m, and b.The equation in "slope-intercept form" would be;

`y=14x+103`

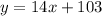
To convert this linear equation into the standard form which is;

`Ax+By=C`

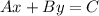
We would move the term with variable x to the left side of the equation;

`\begin{gathered} y=14x+103 \\ \text{Subtract 14x from both sides;} \\ y-14x=103 \end{gathered}`

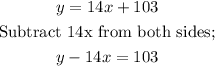
We can now re-write and we'll have;

`-14x+y=103`

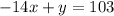
Note that the coefficients of x and y (that is A and B) are integers and A is positive;

Therefore, we would have;

`\begin{gathered} \text{Multiply all through by -1} \\ 14x-y=-103 \end{gathered}`

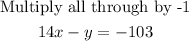
The equation of the line passing through the points given expressed in standard form is;

ANSWER:

`14x-y=-103`