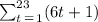Each term is 6 greater than the previous term.
First term is "7".
So,
a = 7
d = 6
Let's find the formula for the series,
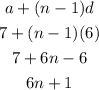
We can immediately eliminate the firsst and third choice.
The variable is "t", so the general formula will be:

How many terms are there?
The series starts from t = 1,
since 6(1) + 1 = 6 + 1 = 7
and 6(2) + 1 = 12 + 1 = 13
The terms match!
So, 2nd answer choice is correct!!
Answer