We need to use some properties of the kyte:
· The opposite obtuse angles are equal. In the figure, this means ∠WZY = ∠WXY
· The large diagonal bisects the angles ∠ZWX and ∠XYZ
56 ask us to find m∠XYZ. We can note that the angles ∠ZXY and ∠XZY are congruent. And we know that the interior angles of the triangle XYZ add to 180º.
m∠VXY = m∠VZY = 58º
Then:
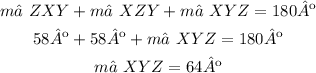
The answer to 56. is 64º
57 ask us to find m∠ZWV, we can use the second property listed above. The large diagonal bisects the angle ∠ZWX. Since we know ∠ZWX = 50º, then:
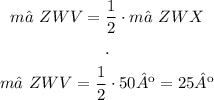
The answer to 57 is 25º
58 ask us to find m∠VZW. We know that the sum of all internal angles of a kite (or any quadrilateral), is 360º.
We know:
m∠ZWX = 50º
m∠WZY = m∠WXY
m∠XYZ = 64º
Then:
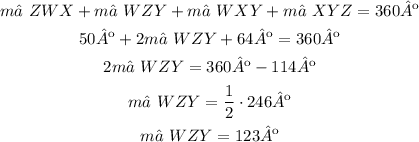
And:
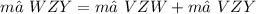
Now replace the known values of m∠WZY = 123º and m∠VZY = 58º:
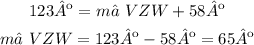
The answer to 58 is 65º
59 ask us to find m∠WZY, we sis it in 58 to find m∠VZW.
The answer to 59 is 123º