It is given that z varies directly with x and inversely with the square of y so it follows:
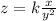
It is also given that z=18 when x=6 and y=2 so it follows:

So the equation of variation becomes:
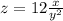
Therefore the value of z when x=7 and y=7 is given by:
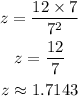
Hence the value of z is 12/7 or 1.7143.