Question

If a seed is planted, it has a 85% chance of growing into a healthy plant 9 seeds are planted, what is the probability that exactly 3 don't grow?

Answer 1

0.1069

Step-by-step explanation

We have two possible outcomes for each experiment: the seed grows or the seed does not grow. So, this follows a binomial distribution, where, in this case, the probability of success is the probability that a seed does not grow - note that we want to find what is the probability that a number of seeds do not grow.

We know that the probability that a seed grows is 85%, so there is a 15% chance the seed does not grow. This experiment is repeated 9 times (9 seeds) and we want to find what is the probability that the number of successes is 3 - remember that "success" is that the seed doesn't grow.

To find this, we have to use the binomial probability formula,

`P(X=x)=\binom{n}{x}\cdot p^x\cdot q^(n-x)`

For this problem:

• n = 9

,

• x = 3

,

• p = 0.15

,

• q = 0.85

So we have,

`P(X=3)=\binom{9}{3}\cdot0.15^3\cdot0.85^6\approx0.1069`

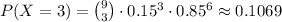
Hence, the probability that exactly 3 seeds don't grow is 0.1069, rounded to four decimal places.