Question

Question 4 of attached screenshot, I have all relevant information if required

Answer 1

The derivative of the function is given as:

`g^(\prime)(x)=(x^2-16)/(x-2)`

It is also given that g(3)=4.

Note that the Slope of a Tangent Line to a function at a point is the value of the derivative at that point.

Substitute x=3 into the derivative:

`g^(\prime)(3)=(3^2-16)/(3-2)=(9-16)/(1)=(-7)/(1)=-7`

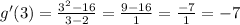
It follows that the slope of the tangent line at x=3 is -7.

Since it is given that g(3)=4, it implies that (3,4) is a point on the line.

Recall that the equation of a line with slope m, which passes through a point (x₁,y₁) is given by the point-slope formula as:

`y-y_!=m(x-x_1)`

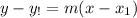
Substitute the point (x₁,y₁)=(3,4) and the slope m=-7 into the point-slope formula:

`\begin{gathered} y-4=-7(x-3) \\ \Rightarrow y-4=-7x+21 \\ \Rightarrow y=-7x+21+4 \\ \Rightarrow y=-7x+25 \end{gathered}`

Hence, the equation of the tangent line to the graph of g at x=3 is y=-7x+25.

The required equation is y=-7x+25.