To answer this question, we can use the standard normal distribution to find the asked percentiles. We will need the z-scores to find the values for them.
The z-scores are given by the formula:

Where
• x is the raw value (the one we need to find here)
,
• μ is the population's mean. In this case, μ = 1.75 Mgal.
,
• σ is the population's standard deviation. In this case, σ = 2.82 Mgal.
Finding the 86th percentile
The 86th percentile represents the value for which 86% of the cases are less than this value, and, therefore, 14% are above this value.
Then we can find it, if we know the value for which, z, in the standard normal table, represents the cumulative probability (0.86) for the distribution. If we consult the table, we have:
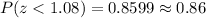
Therefore, the value for z is, approximately, z = 1.08, and we can use this value to find the value for x (the 86th percentile, in this case):
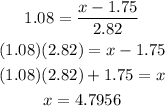
Therefore, the 86th percentile, if we round the value to two decimal places, is, approximately, x = 4.80.
Finding the 28th percentile
We can apply the same procedure as before. Then we have:
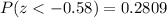
Then we have:
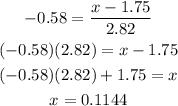
If we round the result to two decimal places, we have that the 28th percentile is x = 0.11 (approximately).
Finding the footprint for the third quartile
The third quartile is equivalent to the 75% percentile. Then we can use the same process as before to find the value of z that represents it as follows:
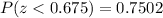
And we can apply the same formula as before:
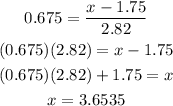
If we round this value to two decimal places, we have x = 3.65 (approximately).