Question

Solve theses equations by elimination y= 3/2x -10 and -2x -4y =-8

Answer 1

We want to solve the question with elimination method

`\begin{gathered} y=(3)/(2)x-10.\text{ . . . . . . equation 1} \\ -2x-4y=-8\text{ . . . . . . . equation 2} \\ multiply\text{ equation 1 by 2, so as to remove the fraction } \\ 2* y=(2*(3)/(2)x)-(2*10) \\ 2y=3x-20 \\ re-arranging\text{ we have } \\ -3x+2y=-20 \end{gathered}`

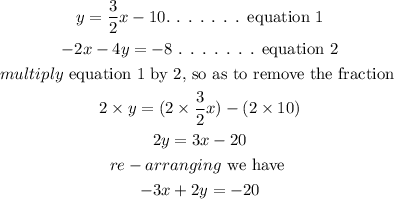
So our paired equation becomes

`\begin{gathered} -3x+2y=-20 \\ -2x-4y=-8 \end{gathered}`

To eliminate y, multiply the upper equation by 4 and the lower by 2, we have

`\begin{gathered} 4(-3x+2y=-20) \\ 2(-2x-4y=-8) \\ -12x+8y=-80 \\ -4x-8y=-16 \\ we\text{ have } \\ (-12x-4x)+(8y-8y)+(-80-16) \\ -16x+0=-96 \\ -16x=-96 \\ x=(-96)/(-16) \\ x=6 \end{gathered}`

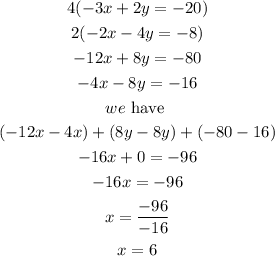
So put x for 6 into the second equation, we have

`\begin{gathered} -2x-4y=-8 \\ -2(6)-4y=-8 \\ -12-4y=-8 \\ -4y=-8+12 \\ -4y=4 \\ y=(4)/(-4) \\ y=-1 \end{gathered}`

Hence x = 6 and y = -1

The graph is shown below

Hence the point of intersection is (6, -1)