Question

The table shows how the number of sit-ups Marla does each day has changed over time. At this rate, how many sit-ups will she do on Day 12? Explain your steps in solving this problem.see image

Answer 1

Maria willdo 61 sit-ups on Day 12

Step-by-step explanation

the table represents a linear function so, we can find the equation of the function and then evaluate for day 12

the equation of a line is given by:

`\begin{gathered} y=mx+b \\ \text{where m is the slope} \\ b\text{ is the y-intercept} \end{gathered}`

so

Step 1

find the slope of the line

the slope of a line is given by.

`\begin{gathered} \text{slope}=\frac{change\text{ in y }}{\text{change in x}}=(\Delta y)/(\Delta x)=(y_2-y_1)/(x_2-x_1) \\ \text{where} \\ P1(x_1,y_1) \\ P2(x_2,y_2) \\ \text{are 2 points from the table } \\ or\text{ 2 coordinates ( from the table)} \end{gathered}`

let

P1(1,17)

P2(4,29)

now, replace

`\begin{gathered} \text{slope}=(y_2-y_1)/(x_2-x_1) \\ \text{slope}=(29-17)/(4-1)=(12)/(3)=4 \\ \text{slope= 4} \end{gathered}`

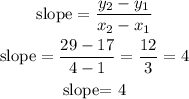
Step 2

now,find the equation of the line,use the slope-point formula

`\begin{gathered} y-y_1=m(x-x_1) \\ \text{where m is the slope} \\ \end{gathered}`

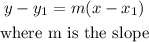
now, replace

`\begin{gathered} y-y_1=m(x-x_1) \\ y-17=4(x-1) \\ y-17=4x-4 \\ y=4x-4+17 \\ y=4x+13 \end{gathered}`

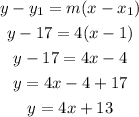
so,the equation of the lines is

y= 4x+13

Step 3

finally, evaluate for day 12, it is x= 12

so,replace

`\begin{gathered} y=4x+13 \\ y=4(12)+13 \\ y=48+13 \\ y=61 \end{gathered}`

it means Maria will do 61 sit-ups on Day 12

I hope this helps you