Question

Can you help me with some math questions the option I chose was troposphere(above the surface

Answer 1

1.

What do you know?

the troposphere extends 8 to 20 km above the Earth's surface. The surface temperature is 15°C and decreases at a rate of 6.5°C per kilometer away.

What do you want to find out?

The temperature at any point of the troposphere

What kind of answer do you expect?

Any value below 15°C

2. Define variables,

T is for temperature, and

d is the distance away from the surface

3. What is the b -value

b = 15

which is the Earth's surface temperature or the initial temperature for our problem

4. What is the slope

the slope 'm' equals -6.5

which means that the temperature decreases 6.5°C per km

5. write the equation

`\begin{gathered} y=b+mx \\ \Rightarrow T=15-6.5d \end{gathered}`

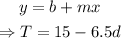
the general form for this equations is y = b + mx

thus, for our problem, the equation is: T = 15 - 6.5*d

6.

a) the slope represents the rate of change of temperature with respect to distance, since m = -6.5 °C/km , this means the troposphere is getting 6.5 °C colder per km away from Earth's surface

b) the y-intercept represents the Earth's surface temperature

Graph:

7. identify 3 points

when d = 0 then T = 15, so our first point is (0,15)

when d = 1 then T = 15 - 6.5 = 8.5 , so our second point is (1,8.5)

when d = 2 then T = 15 - 13 = 2 so our third point is (2,2)

8. calculate the slope

let's use this equation and this set of points,

`\begin{gathered} m=(y2-y1)/(x2-x1) \\ (x1,y1)=(1,8.5) \\ (x2,y2)=(2,2) \end{gathered}`

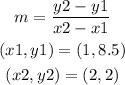
let's replace and solve

`m=(2-8.5)/(2-1)=(-6.5)/(1)=-6.5`

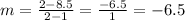
We can see that it does match the slope from our model, which is -6.5

9. graph the line and the points,

10. Complete the sentence

... ends between 8 km and 20 km above Earth.

11. temperature at the farthest point

when d = 20 then,

`\begin{gathered} T=15-6.5*20 \\ =15-130 \\ =-115 \end{gathered}`

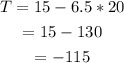
... the temperature is -115°C

12. Temperature range,

from... 15 °C to -115 °C