Question

The figure below shows a circular lawn. It’s diameter is 72 ft.a.Use 3.14 for n in your calculations,and do not round your answer.Make sure to include the correct units.B.Which measure would be used in finding the amount of fertilizer needed? C.Which measure would be used in finding the amount of tape needed?

Answer 1

Answers:

a) Area = 4069.44 ft²

Circumference = 226.08 ft

b) Area

c) Circumference

Step-by-step explanation:

The area of the circular lawn can be calculated as:

`\text{Area}=\pi\cdot r^2`

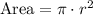
Where π is 3.14 and r is the radius of the circular lawn.

The radius is half the diameter, so the radius is equal to:

`r=\frac{\text{Diameter}}{2}=\frac{72\text{ ft}}{2}=36\text{ ft}`

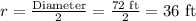
Then, the area of the lawn is equal to:

`\begin{gathered} \text{Area = 3.14}\cdot(36ft)^2 \\ \text{Area}=3.14(1296ft^2) \\ \text{Area}=4069.44ft^2 \end{gathered}`

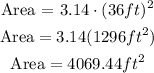
On the other hand, the circumference of the lawn can be calculated as:

`\text{Circumference = 2}\cdot\pi\cdot r`

So, the circumference is equal to:

`\begin{gathered} \text{Circumference = 2}\cdot\text{(3.14)}\cdot(36\text{ ft)} \\ \text{Circumference = }226.08\text{ ft} \end{gathered}`

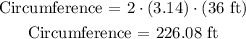
Finally, the fertilizer is applied to the region, so the measure that you would use to find the amount of fertilizer is the area.

In the same way, to surround the lawn, the measure that would be used to find the amount of tape is the circumference.

So, the answers are:

a) Area = 4069.44 ft²

Circumference = 226.08 ft

b) Area

c) Circumference