Question

A) calculate the extension of wire needed to produce this tensionBCalculate the work done in producing this extension

Answer 1

Given,

The length of the string, L=0.85 m

The diameter of the string, d=6×10⁻⁴ m

The radius of the string, r=3×10⁻⁴ m

The Young's Modulus of the string, Y=190 GPa

The tension, F=66 N

a)

The Young's Modulus is given by,

`Y=(FL)/(Al)`

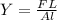
Where A is the area of cross-section of the string and l is the extension produced in the string.

On rearranging the above equation,

`l=(FL)/(YA)`

On substituting the known values,

`\begin{gathered} l=(66*0.85)/(190*10^9*\pi(3*10^(-4))^2) \\ =1.04*10^(-3)\text{ m} \end{gathered}`

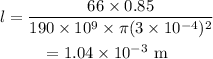
Thus the extension of the wire that is needed to be produced is 1.04×10⁻³ m

b)

The work done in producing the extension is given by,

`W=(1)/(2)Fl`

On substituting the known values,

`\begin{gathered} W=(1)/(2)*66*1.04*10^(-3) \\ =0.034\text{ J} \end{gathered}`

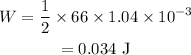
Thus the work done in producing the extension is 0.034 J