we are given a proportional relatrelationshipioship and the following statements:
1. 2.5 pounds of peanuts cost $1. To answer this statement we need first to find the equation of the line that models the problem. Since the relationship is proportional the equation is of the form:

Now we replace the given point, that is: (7, 17.5), this means that when x = 7, y = 17.5. Replacing we get;

Solving for "m":
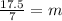
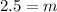
Replacing in the equation we get:

Now we replace the value of x = 2.5 pounds we get:
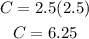
Therefore, 2.5 pounds cost $6.25. The statement is false.
2. 1 pound costs 2.5. replacing x = 1 we get:
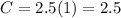
Therefore, 1 pound costs $2.5. the statement is true
3. 5 pounds of peanuts cost 12.5. Replacing x = 5
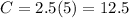
Therefore, 5 pounds cost 12.5. The statement is true:
4. 9 pounds cost 19.5. Replacing x = 9, we get

Therefore, 9 pounds cost 22.5. The statement is false.
5. The point (4,10) is on the graph. To determine this we need to replace x = 4, if we get 10, then the statement is true. Replacing x = 4 we get:
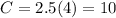
Since we got 10, the statement is true.