Given:
The sum of the speeds of two trains is 721.4
Let the speed of the trains are x and y
So,
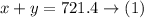
And the speed of the first train is 4.6 mph faster than the second train
So,
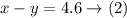
Solve the equations (1) and (2) to find x and y
Add the equations to eliminate (y) then solve for (x):
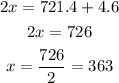
Substitute (x) into the first equation then solve for (y):
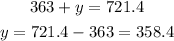
So, the answer will be:
The speeds of the trains are 363 mph and 358.4 mph