The exterior and interior angles of a polygon are supplementary angles (they add up to 180 degrees). Since the exterior angle of the polygon has a measure of 10 degrees, then the interior angles must have a measure of 170 degrees.
On the other hand, the measure of the internal angle of an n-sided polygon is:
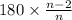
Since the internal angle must have a measure of 170 degrees, then:
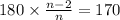
Solve for n:
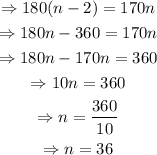
Therefore, the polygon has 36 sides.