Answer:
1) 329 grams
2) 45 days
Explanations:
The function representing the the number of grams present after t days is:
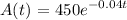
To find the number of grams left after 8 days, substitute t = 8 into the function above:

A(8) = 329 (to the nearest whole number)
329 grams are left after 8 days
2) How long until 75 grams are left
That is, A(t) = 75
To get the value of t for A(t) = 75
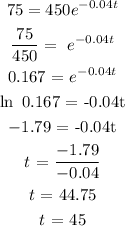
It will take 45 days for 75 grams to be left