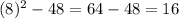Question

There is a parallelogram ABCD. E is the internal center point of the parallelogram. Point A to point E is 16. Let BE= x sq - 48 and DE 2x. What is the length of BE and DE?

Answer 1

WE are given the following

We are told that AE is 16 and that E is the cneter of the parallelogram. The center of the parallelogram has the property that it splits each diagonal into two line segment with the same length. We are told that the length of segment BE is x²-48 and the length of ED is 2x. Thus, we have the following equation

`x^(2)-48=2x`

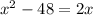
which is equivalent to

`x^(2)-2x-48=0`

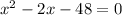
Note that given the polynomial of the form

`ax^(2)+bx+c=0`

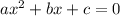
we have that the solutions would be

`x=\frac{-b\pm\sqrt[]{b^(2)\text{ -4ac}}_{}}{2a}`

In our case, we have a=1, b=-2 and c=-48. Thus The solutions would be

`x=\frac{-(-2)\pm\sqrt[]{(-2)^2-4\cdot(-48)}_{}}{2}=\frac{2\pm\sqrt[]{4+192}}{2}=\frac{2\pm\sqrt[]{196}}{2}=(2\pm14)/(2)`

So the solutions would be

`x_1=(2+14)/(2)=(16)/(2)=8`

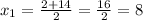
and

`x_2=(2-14)/(2)=(-12)/(2)=-6`

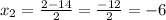
Since DE has length 2x and this number should be positive, we must have that x=8. So, the length of DE would be

`2\cdot8=16`

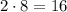
and thus, the distance BE is

`(8)^2-48=64-48=16`