Step 1: Determine if if the infinite geometric series is divergence or convergence
If the common ratio is greater than or equals 1, then it is divergence but if r is less than 1, it is convergence.
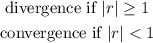
Step 2: Find common ratio r
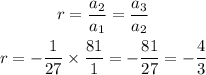
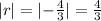
From the value of the absolute value of r gotten, it can be observed that r is greater than 1 or equals 1, hence it is divergence.
Hence, the series will have an infinitely large sum. The correct option is FALSE