Given:
R1 = 3 Ω
R2 = 6 Ω
R3 = 8 Ω
V = 24 v
To find:
a) The equivalent resistance of the system.
b) The potential drop across each resistor.
c) The current through each of the resistors.
Step-by-step explanation:
a)
The equivalent resistance of the circuit can be calculated as:
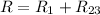
Here, R23 is the parallel equivalent resistance of resistors R2 and R3.
Substituting the values in the above equation, we get:
![\begin{gathered} (1)/(R_(23))=\frac{1}{6\text{ \Omega}}+\frac{1}{8\text{ \Omega}}=\frac{8}{8*6\text{ \Omega}}+\frac{6}{6*8\text{ \Omega}}=\frac{14}{48\text{ \Omega}} \\ \\ R_(23)=\frac{48\text{ \Omega}}{14}=3.43\text{ \Omega} \end{gathered}]()
The equivalent resistance of the system is given as:
![R=R_1+R_(23)=3\text{ \Omega}+3.43\text{ \Omega}=6.43\text{ \Omega}]()
b)
The current through the circuit can be calculated by using Ohm's law.
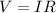
Substituting the values in the above equation, we get:
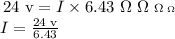
As the current flowing through each resistor in a series combination is equal. The current through R1 and R23 (the parallel equivalent of R2 and R3) is 3.73 A.
Now, the potential drop V1 across each resistor R1 is calculated as:

The potential drop V23 across R23 is calculated as:
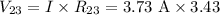
In a parallel combination of resistors, the potential drop across each resistor is the same. Thus the potential drop across resistor R2 and R3 is 12.79 v.
c)
The total current in a parallel circuit is the sum of currents through individual resistors. As the voltage drop across each resistor in parallel combination is the same, the current I2 through resistor R2 and I4 through resistor R3 can be calculated as:
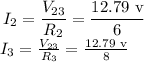
Final answer:
a) The equivalent resistance of the system of resistance in the given circuit is 6.43 Ω.
b) The potential drop across resistor R1 is 11.19 v, the potential drop across resistor R2 is 12.79 v and the potential drop across resistor R3 is 12.79 v.
c) The current through resistor R1 is 3.73 A, the current through resistor R2 is 2.13 A and the current through resistor R3 is 1.60 A.