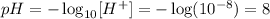REmember that pH is the negatige logarithem of the concentration of protons:
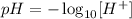
when we make the invers of the logarithm:
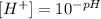
in this case:
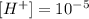
if the concentration of protons undergoes a 1000-fold increase the new concentration is:
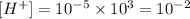
therefore the new pH is calculated as follows:
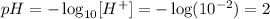
B.
For the second part we need to remember that [H+] and [OH-] are related according the following equation:

We have calculated before that
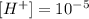
Then we can calculate [OH-]:
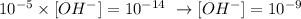
1000 fold that concentration is
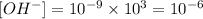
Again we use the relation between [H+] and [OH-] with the new value:

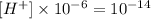
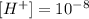
And once again the pH formula: